Zonohedra

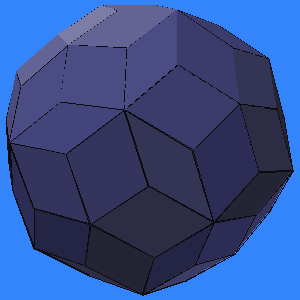
    A zonohedron (by one restrictive definition) is a convex polyhedron all of whose faces are parallelograms. (less restrictive definitions, below, allow other types of faces.) You can get a feel for them by exploring and comparing a "random" zonohedron with 42 faces and this nicely structured rhombic enneacontahedron, shown at right, a zonohedron with 90 faces. In the first case there are 21 different "random" parallelogram shapes for the faces. In the second case there are only two different shapes, and they are both rhombic, with the same edge length. (And in case you missed it, "enneaconta" means "90".)

    The faces of a zonohedron can be grouped into zones. A zone is an encircling band of faces which share a common edge direction (and length). This is best understood by examining a model of the same "random" 42-face zonohedron as above, or a rhombic enneacontahedron, or a rhombic tricontahedron, in which one zone is colored. Each face is part of two zones. Because opposite edges of each parallelogram are parallel, the faces can be grouped into chains which all share a common direction in space. The chains must be closed cycles because there are only a finite number of faces.

    Exercise: Look and count to see that each zone in the random 42-face zonohedron contains 12 faces, and that each zone in the rhombic enneacontahedron contains 18 faces. Also observe that any pair of zones intersect each other in exactly two places --- at two opposite equal faces.

    Three important zonohedra are the cube, the rhombic dodecahedron, and the rhombic triacontahedron.

    Exercise: Count and see that these three zonohedra have 3, 4, and 6 zones, respectively. The zones consist of 4, 6, and 10 faces respectively.

    These three zonohedra are closely related to the Platonic solids:

    • The diagonals of the faces of a cube form two tetrahedra.
    • The short diagonals of the rhombic dodecahedron outline a cube, and the long diagonals outline its dual octahedron. Here is a model of the cube and the octahedron inscribed in the rhombic dodecahedron, and here is a model of just the edges of all three together.
    • The long diagonals of the rhombic triacontahedron outline an icosahedron, and the short diagonals outline its dual dodecahedron. Here is a model of the dodecahedron and the icosahedron inscribed in the rhombic triacontahedron, and here is a model of just the edges of all three together.
    By stretching and skewing the cube, one can more generally obtain simple zonohedra called parallelepipeds, having six parallelogram faces in three equal opposite pairs.

    Observe that the zonohedra above have 6, 12, 30, 42, and 90 faces. Hindsight indicates that these numbers are of the form n(n-1) where n is 3, 4, 6, 7, or 10. This should suggest the case of n=5.

    Exercise: Can you make a zonohedron with 20 sides? In other words, set n=5 in the formula n(n-1).
    Hint: Study the n=6 solution, the rhombic triacontahedron.
    Answer: Work on it before looking at the answer!

    The number n is just the number of zones, and each pair of zones meets twice (once in front and once in back). There are n(n-1)/2 possible pairs we can choose from n zones, and so n(n-1) faces.

    We can create a zonohedron from any set of three or more vectors or directions in space. Three vectors define a parallelepiped. Adding an additional vector to the set leads to enlarged zonohedra (with one more zone) by the reverse of the 6-zone-to-5-zone process referred to above.

    To construct symmetric zonohedra by this process, we start with a symmetrically arranged set of vectors, for example the axes of symmetry of a symmetric polyhedron. These directions in space form what is called a star of vectors. Every zonohedron has an underlying star---its edge directions---and from any star we can construct a zonohedron having those edge directions:

    • Using the diagonals of the octahedron (3 mutually orthogonal vectors) as the star, we construct the 3-zone cube.
    • Using the diagonals of the cube (4 vectors in the directions of the vertices of the tetrahedron) as the star, we construct the 4-zone rhombic dodecahedron.
    • Using the 6 diagonals of the icosahedron as the star, we construct the 6-zone rhombic triacontahedron.
    • Using the ten diagonals of the dodecahedron as the star, we construct the 10-zone rhombic enneacontahedron, shown above.
    • Using a set of vectors positioned like the ribs of an umbrella, we get a polar zonohedron. Here is an example 20-zone polar zonohedron. The rhombic icosahedron is a 5-zone polar zonohedron.
    Five of the zonohedra listed above have a single kind of rhombic face, and are duals to quasi-regular polyhedra with vertices of types (m, n, m, n). For example, the rhombic triacontahedron is dual to the icosidodecahedron, with vertices of type (3,5,3,5). Looking at their compound shows this relationship nicely.

    There are several ways to generalize the above notion of zonohedron. One is to allow nonconvex polyhedra; a second is to allow polygons with more than four sides. A third is an original concept which I call a zonish polyhedron, in which an arbitrary polyhedron is used as a seed:

    • Two interesting nonconvex zonohedra are the medial rhombic triacontahedron and the great rhombic triacontahedron. These are both stellations of the rhombic triacontahedron.
    • If a given star contains three or more vectors in a plane several parallelogram faces will lie in the same plane and join to form a larger centrally symmetric polygon. Here is a cute example of a 36-zone zonohedron with 864 rhombi and six 24-sided faces. If you like it, check out the zonohedrification page.
    • Although I have not seen this described in print anywhere, we can easily apply the zone addition process to an arbitrary seed polyhedron. This leads to a wide range of interesting structures which I call zonish polyhedra.
    Here is a list of some zonohedra, along with their duals, and their compounds.

    Good references on zonohedra are W.W. Rouse Ball, Mathematical Recreations and Essays, p. 140, and Coxeter, Regular Polytopes, p. 27.

    Virtual Polyhedra, (c) 1996, George W. Hart