Prisms and Antiprisms

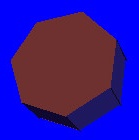    There are two classes of polyhedra which belong with the Archimedean solids, but are usually listed separately because they form an infinite sequence. Both classes are suggestive of a drum with string tying together the top and bottom membranes. The top and bottom can have any number of sides. The two classes differ according to whether the strings go up-and-down or zig-zag,

    A prism consists of two copies of any chosen regular polygon (one becoming the top face, and one becoming the bottom face), connected with squares along the sides. By spacing the two polygons at the proper distance, the sides consist of squares rather than just rectangles. At each vertex, two squares and one of the polygons meet. For example, based on a 7-sided heptagon, is the heptagonal prism (4,4,7).

    An antiprism also consists of two copies of any chosen regular polygon, but one is given a slight twist relative to the other, and they are connected with a band of alternately up and down pointing triangles. By spacing the two polygons at the proper distance, all the triangles become equilateral. At each vertex, three triangles and one of the chosen polygon meet. Example: heptagonal antiprism. (3,3,3,7).

    I have included models of prisms and antiprisms ranging from three sides up to ten sides in this list of prisms and antiprisms.

    A special case of the prism is the cube (when the chosen top and bottom face is a square). And, a special case of the antiprism is the octahedron (when the chosen top and bottom face is a triangle).

    Kepler was the first to discuss antiprisms, and recognize the connection between prisms and the Archimedean solids.

    Virtual Polyhedra, (c) 1996, George W. Hart