The Great Disnub Dirhombidodecahedron
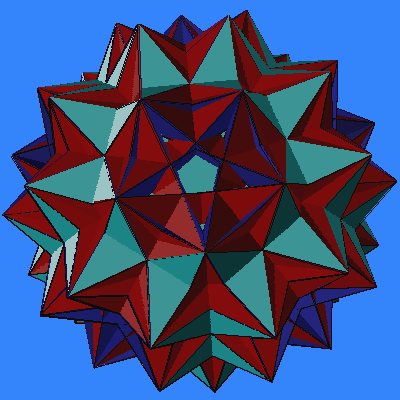This
page describes a unique polyhedron, first described by J.
Skilling in 1975, which he named the great
disnub dirhombidodecahedron. In deriving this figure, Skilling considered
an interesting generalization the usual definition of polyhedron. This
object has four faces meeting at some of its edges, while the usual definition
of a polyhedron requires that exactly two faces meet at each edge. In all
other respects, it satisfies the definitions of the nonconvex
uniform polyhedra: it is composed of plane regular polygons, with the
same arrangement of faces at each vertex. Remarkably, although there are
75 uniform polyhedra plus the infinite series of prisms and antiprisms,
when one broadens the definition to allow any even number of faces to meet
at each edge, Skilling's computer search showed that only this one new
object occurs.
The great disnub dirhombidodecahedron has 60 vertices and 204 faces. (There are 120 triangles, 60 squares, and 24 pentagrams. The squares all pass exactly through the center, and the pentagrams come in twelve coplanar pairs.) It has 240 edges. (At 120 edges, two faces meet; at the other 120, four faces meet. At each vertex, twelve faces meet, but only eight edges.) It is closely related to a number of other polyhedra, particularly the great dirhombicosidodecahedron which has the same vertices and edges, and the same squares and pentagrams, but its 40 triangles are different. It is also related to the compound of twenty octahedra, which also has the same vertices and edges, and includes among its 160 triangles the 120 triangles of the great disnub dirhombidodecahedron. (The 60 squares are the "equatorial" squares of these octahedra.) In fact, to construct this great disnub dirhombidodecahedron model, I effectively took the exclusive-OR of the great dirhombicosidodecahedron and the compound of twenty octahedra, i.e., any face which is in one or the other of these, but not both.
Also related are the compound
of 20 tetrahemihexahedra, and the great
snub dodecicosidodecahedron which both have the same vertices and share
certain faces.